Koch Flake

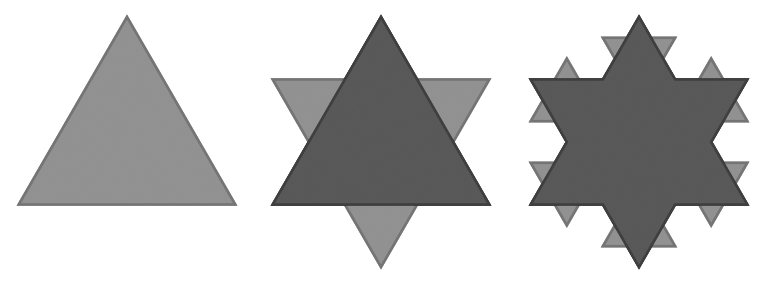
The Koch snowflake is a fractal curve obtained by starting with an equilateral triangle and recursively adding equilateral triangles to the center of each side of the initial triangle. The resulting pattern resembles a snowflake and exhibits self-similarity at different scales. It was first described by the Swedish mathematician Helge von Koch in 1904.

To draw the Koch snowflake, follow these steps/algorithm:

1 - Draw an equilateral triangle (made up of 3 equal segments)

2 - Divide each segment into three equal sub-segments

3 - Construct an equilateral triangle with the central sub-segment as its base and pointing outwards.

4 - Delete the central sub-segment

5 - Repeat steps 2 to 4 for each of the segments of the new figure obtained

The perimeter of the Koch snowflake increases with each iteration. For an initial triangle with side length $ L $, the general formula for the perimeter after $ n $ iterations is: $$ 3 L \left( \frac{4}{3} \right)^n $$

Indeed, the initial triangle has an initial perimeter of $ 3L $. At each iteration, any segment composed of 3 sub-segments is replaced by a broken line of 4 segments (all of the same size). The number of segments is therefore multiplied by $ 4/3 $ at each step. > After 2 iterations, a line of initial length $ L $ is now $ L \times \frac{4}{3} \times \frac{4}{3} = L \times \frac{16}{9} \approx 1.778L $

When $ n $ tends to infinity, then $$ \lim\limits_{n \to +\infty} 3 L \left( \frac{4}{3} \right)^n = +\infty $$

The total length of the fractal curve is therefore theoretically infinite.

With an initial triangle of side length $ L $, the formula for the total area of the snowflake after $ n $ iterations is:

$$ A_n = \frac{\sqrt{3}}{20} L^2 \left( 8-3 \left( \frac{4}{9} \right) ^n \right) $$

As $ n $ approaches infinity, the area of the snowflake converges to a finite value:

$$ A = \lim\limits_{n \to +\infty} A_n = \frac{ 2 \sqrt{3} }{5} L^2 $$

This area is exactly $ 8/5 $ of the area of the initial triangle (which was $ \frac{\sqrt{3}}{4} L^2 $)

The fractal dimension of the Koch snowflake, denoted $ D $, is calculated using the fractal dimension formula: $$ D = \frac{ \ln(3) } { \ln(4) } \approx 1.2619 $$

The Koch snowflake can be seen as a fractal attractor.

The number of segments composing the snowflake after $ n $ iterations is given by the formula: $$ 3 \times 4^n $$

Each segment decreases in size at each iteration according to the formula $ L_{n} = \frac{L}{3^n} $

It is impossible to construct a Koch snowflake in the real world perfectly because it would require an infinite number of subdivisions.

However, it is possible to approximate the final curve with a large number of iterations, making the result visually very close to the theoretical snowflake.